CC0 http://pixabay.com/en/sunset-boat-sea-ship-675847/
The lecture, textbook, worksheet, pop-quiz, test and exam cycle are the traditional delivery tools used in most schools to teach mathematics. It’s the way you and I were probably taught math. Teachers, at the helm of this tightly run ship, lead their crew through the abstract terrain of formulas, equations, rules and processes in a very linear flow, with a strict time frame decided by the publishing company and the curriculum. We’re told that it has ‘worked’ for years. But what does this method do for the joy of sailing, for the exploring of unchartered territory, for discovering new lands?
Teachers report a lack of engagement in the wonder of problem solving on the part of many of their students. This valuable application skill of math has caused a parting of the seas of sorts, whereby those few students that “get it” sail off into the abstract, while the majority who need more interactive measures are thrown to the sharks!
To encourage students to be more patient and resilient problem solvers, there has to be a better trajectory for mathematics instruction, with varied and exciting techniques and strategies that are more intuitive and applicable to the real world. Much research has been done over the years to get students to love and succeed in math, but our industrial model for mathematical instruction runs deep and teachers are wary of what they see as flavour of the month strategies that so often disappoint.
Provincial exams don’t help in righting the course, often contributing to the “drill and kill” mentality which in turn shackles educators to a rigid curriculum that revolves around textbooks, workbooks, practice sheets written by publishing houses intent on providing teachers with a direct line to mathematics instruction, taking the soul and wonder out of its’ sails.
Can we right this ship?
Lost at sea: Student anxiety
This sense of being lost at sea is discussed by Matthew A. Brenner in his essay The Four Pillars Upon Which the Failure of Math Education Rests (and what to do about them). Math instruction suffers from “the long-term, chronic frustration and shame inflicted on countless millions of students for years on end in the name of math education.” In elementary school, students start to experience a sense of being lost, overwhelmed, confused and flounder in the tidal wave of math when taught in a rote manner. Math anxiety even has its own Wikipedia entry! Brenner believes understanding must be front and centre for “where there is not understanding there can only be the most shallow learning: mindlessly memorizing facts and procedures… all that remains are bits of unconnected specific information: the multiplication table, a formula for variance, another for standard deviation, etc.”
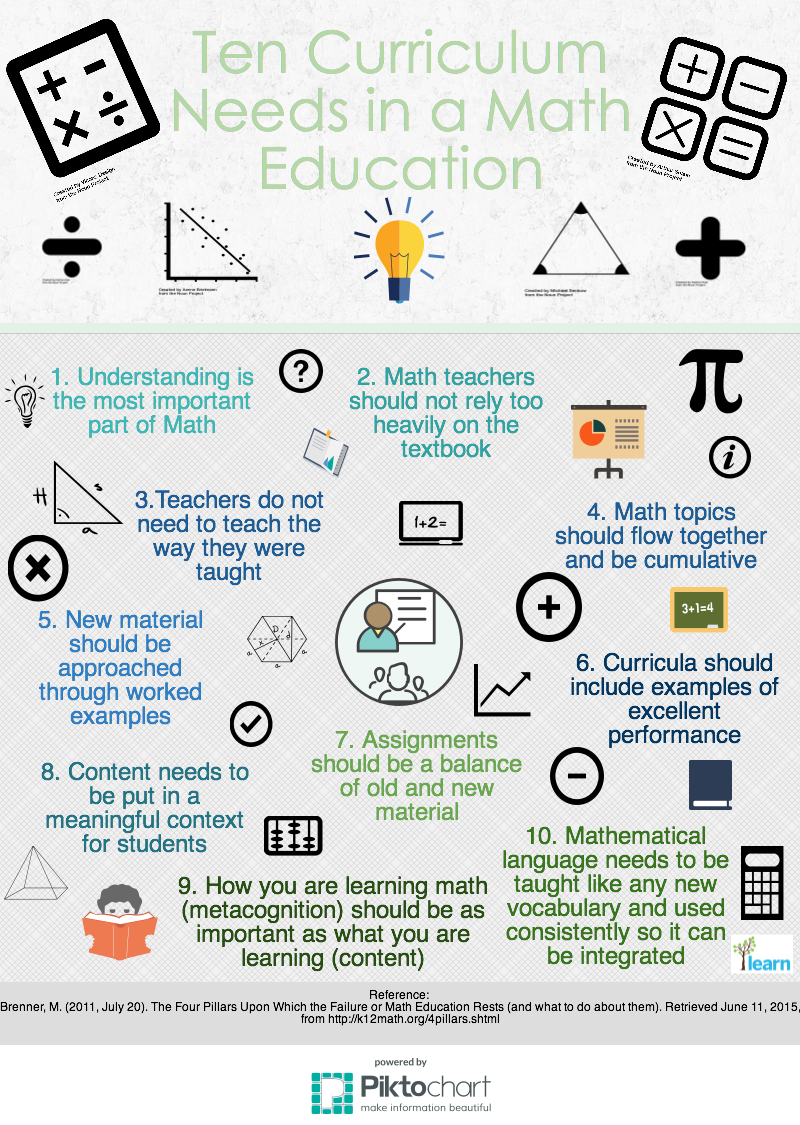
The following table illustrates how educators might begin to right this ship.
A focus on understanding
Understanding is paramount to the development of math competency. For example, learning fractions without knowing that a fraction is a piece of one whole thing is a waste of time, and confusing. What is memorized is not learned. How big can the pieces of your birthday cake be if you have eight guests? Hmmmm. Deep learning requires a lot of varied input and cognitive engagement from baby steps in recognition until the concept is learned. Far too often math textbooks present abstract and disconnected topics in a constricted time frame with examples that don’t inspire our students to want to learn more. Teachers worried about falling behind, skip crucial steps to understanding due to perceived time constraints.
Bewildered students end up fearing what lies ahead as they struggle to memorize disconnected bits. Heads down, they see the mop swabbing the deck, bits of rope and sail, and do not notice the sea, sunsets and seaspray on their skin.
If understanding and connecting topics is what we truly seek for our learners, then instruction must involve a variety of practical resources, hands-on experiences, meaningful discussion about math that cause students to engage and reflect on concepts, to use understanding to build new understanding. Equipped with basic understanding of math concepts, and ongoing collaboration opportunities with peers and of course fun, problem-solving strategies will evolve naturally…
…information is an undigested burden unless it is understood. It is knowledge only as its material is comprehended. And understanding, comprehension, means that the various parts of the information acquired are grasped in their relation to one another—a result that is attained only when acquisition is accompanied by constant reflection upon the meaning of what is studied. – John Dewey (1933)
We must stop teaching math the way we learned math. There is enough data that shows that how we learned math did not work for the majority of our students. If cumulative understanding is our ultimate goal, our curriculum needs a gutting. Concepts have to be understood first, no matter how long that takes, nor how many different ways to that goal are needed! Thus acquired learning can be built upon, with the big picture always guiding our course!
For such deep understanding to happen, using worked examples and their solutions (clearly laid out examples that are relevant and applicable in a context that the students can relate to, like birthday cake and sunsets) when introducing new material helps. This will lower the cognitive load on the student and provide a foundation of procedures for solving situational problems for the future. Take the following example from a grade 9 textbook;
In a bicycle race, Lionel gives Robert a 500 m advantage. Also, Lionel agrees to start 15 min after Robert. If Lionel bikes at 17 km/h and Robert at 14 km/h, how long will it take Lionel after he starts biking to overtake Robert? (Brown, Dolciani, Sorgenfrey, & Kane, 2000, p.53, problem 20).
Why give a lead in a race? Where’s the finish line? What about hills? Adrenaline in the bikers? Traffic lights? Truly void of reality, context, logic… we ask our students to make sense out of nonsense.
Encouraging students to use past understanding to build new understanding is essential in all assignments. Meaning is what is remembered, not specific detail: “New concepts and techniques should be well-grounded in concrete contexts, until they are well understood and practiced, at which point it is appropriate to discuss and apply them in more abstract and decontextualized ways.” (Brenner, 79) Thus problems like the one above can be exciting as an application of our understanding, like crossword puzzles or sudoku… exercise for the brain. Unengaged students, students who lack basic understanding of mathematical concepts will get no satisfaction, feel stupid or unhappy and jump ship… missing out on a wonderful journey from which they will not easily recover.
Cognitive and metacognitive thinking is inherent in all mathematical activity. Brenner explains it best as, “recalling information and ideas that may be helpful in solving a problem is a cognitive activity, while monitoring the progress and managing the process of solving a problem are metacognitive activity” (Brenner, 60). Students need to develop their deep processing skills in math, which can only be done by doing it. Keep the math language simple and consistent. Avoid using elaborate terms, sentence structures and complex language.
Murky math seas can be calmed if teachers’ ultimate aim is understanding, with a keen eye on the big picture. The more we understand, the more sense the world makes. So all aboard, let’s leave our old ports for seas of deep blue understanding! And cake and sunsets.
*******
Brenner, M. (2011, July 20). The Four Pillars Upon Which the Failure or Math Education Rests (and what to do about them). Retrieved June 11, 2015, from http://k12math.org/4pillars.shtml
Dolciani, M. (1986). Algebra and trigonometry: Structure and method (Vol. II). Boston, Massachussets: Houston Mufflin.