I was a rule-follower as a student. I understood, and eventually mastered, the whole studenting game. Do what you’re told, follow the algorithms, remember all the things forever, and you’ll get good marks. I enjoyed following those algorithms because of the security they offered – I knew I’d get the right answer. Sometimes I understood how the algorithms worked, for example, why we needed to have common denominators to add fractions, and I basked in that understanding. But I definitely did not understand why multiplying fractions worked, or what partial fractions even were once I got to CEGEP. I had no idea why the prescribed procedure worked, only that it did, and that was good enough for me.
I loved math and math class, and was “successful” by today’s questionable standards, but I can’t help but wonder what might now be different if I’d had the opportunity to play in math class. To this day, I shy away from problem-solving, or anything unfamiliar, especially in public spaces. If I don’t already know how to do it, I don’t do it. And I’m a math teacher who loves math!
I am convinced that the lack of play and fun and experimentation in those math classes did as much damage to “strong” students as to those who struggled with math. We all needed the opportunity to think for ourselves, and to experience success as a result. How else could we become resilient, confident, creative people? For this reason, I have tried hard to create for my own students a very different math class experience than mine was.
What do I mean by playing in math class?
I mean pretty much the same thing as regular playing. It can be a game, or not. It could be anything that we get drawn into, as opposed to pushed. It is fun, maybe challenging, organic, non-threatening, and fluid. It can look different on different days, some days in groups, sometimes individually. There is some structure, maybe a common goal, but with more than one way to reach it. Or it might involve creativity, like drawing or building something.
Competition doesn’t need to be involved either, in fact, competition can cause some kids to tune out. I know that student-Audrey would immediately check out if there was going to be a winner and a loser. In fact, competition for marks is often the reason many students check out of math in the first place. I’m not saying there is no place for competition in math play, but it could negatively impact how safe some students feel.
The case for play
As a teacher, I know there is always so much pressure to cover the curriculum in time, so where would this time for play suddenly come from? The case for playtime is much the same as that made for problem-solving by Peter Liljedahl in Building Thinking Classrooms in Mathematics. His research indicates that giving students “non-curricular tasks” to serve “as a primer for the curriculum-driven tasks” actually improves outcomes. His research also found that the time spent on these non-curricular tasks was actually more than made up for by stimulating students to think more and think better while performing traditional curricular tasks. (Liljedahl, 2020)
Who is playing
Note that I don’t just mean that the students should get to play – the teacher should too, otherwise the message is once again, you are here to follow my rules, we are different you and I, I have already learned this, now it’s your turn. When the teacher plays alongside the students it sends the message – all humans of all ages like and deserve to have fun and it is good for us.
Since I’m advocating that this all happen in the classroom, I want to address what are likely some understandable concerns about how to manage playing during class in real time. In two words: Desmos activities. They are designed to greatly facilitate this – teachers can see what everyone is doing and even communicate privately from their computer while the playing is happening.
Actual examples
But of course this is math class so ideally there needs to be some math involved! Here are a few concrete ways I’ve introduced playing in math class.
Desmos Polygraph
If you are unfamiliar with Desmos activities, you have only to go to teacher.desmos.com to discover them, but for now, Desmos has a great game called Polygraph that is built right into the platform. There are lots of Polygraph games that are already made and ready to use but it’s very easy for a teacher to create their own customized polygraph game. The game takes place entirely online, but can happen in either a synchronous online class or in a f2f classroom.
Here’s how the game works:
Students enter the game via a link, and once they’re in, they are randomly paired with another student. One student is then chosen to be the “picker” and the other is then the “guesser”. Both students then see 16 tiles for Ice cream tiles by Alice Keeler or triangles by Noel Hayden
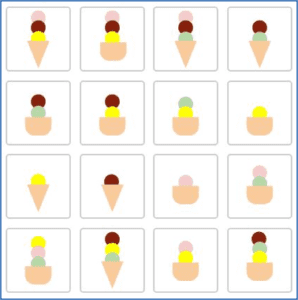
Ice cream tiles by Alice Keeler
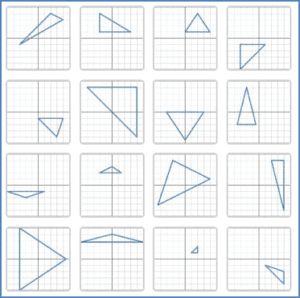
Triangles by Noel Hayden
The picker selects one of the tiles, but their choice is known only to them. The guesser is then prompted to ask the picker yes-or-no questions in order to be able to eliminate tiles, and eventually identify the selected tile. The cycle is then repeated with new pairs of students. Notice that the tiles don’t necessarily have to be mathematical in nature.
What typically happens during successive games is that the questions tend to get more and more specific, vocabulary-rich and even insightful. While it’s not inherently a competitive game, it often became so in the sense that teams derive a great deal of pride for getting it in the least number of questions.
My students enjoyed these games very much, and they often kept playing after class was done. More importantly, it organically opened the door to discussions on all manner of things, eg properties of triangles, even logic. For example, when a guesser asks” Is the triangle a non-right triangle”, and the picker answers no, leads to the meaning of a double negative. These discussions also can happen in real time thanks to the Desmos teacher dashboard, from which the teacher can control the pace and select students’ words (anonymously if desired) to prompt discussions.
But playing doesn’t always have to involve a game – it can just be creating things and trying things out without risk of “getting it wrong”
Polypad (by Mathigon) is an astonishingly rich platform for not only games but math-inspired art, in which students can draw entirely virtually. Like Desmos, there are ready-made activities as well as built-in tiles to allow teachers to create customized activities. For example, students can rearrange, copy, and rotate tangram tiles:
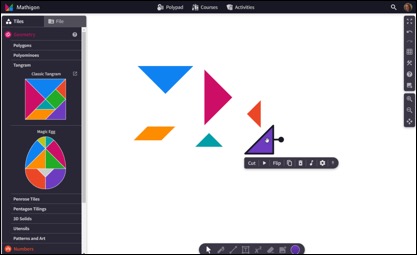
to create their own shape
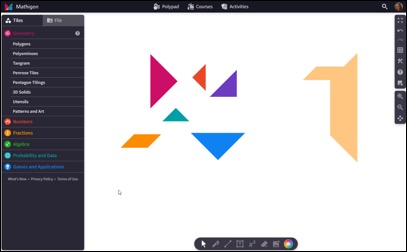
or try to match a given shape
Thanks to a recent merger between Desmos and Mathigon, activities such as this (as well as penrose tiles, tantrix tiles, and fractals, to name a few) can now be embedded within a live Desmos activity so that the teacher can see from the teacher dashboard what each student is doing, as well as share (again, anonymously if desired) students’ creations or solutions.
Marbleslides
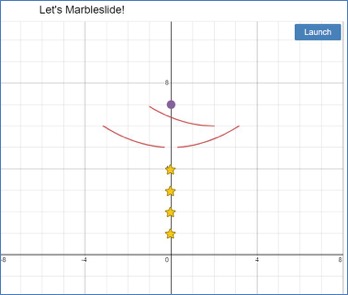 Another great opportunity for play is Desmos’ is Marbleslides. Like the Polygraph game, there are many Marbleslides games already available but teachers can easily create their own as well.
Another great opportunity for play is Desmos’ is Marbleslides. Like the Polygraph game, there are many Marbleslides games already available but teachers can easily create their own as well.
At right is an example of a screen from a Marbleslides game. When the “launch” button is clicked, the “marbles” (represented by the purple circle) fall, and their path is guided by the red curves. The object is to make the marbles touch all of the stars.
In the first example, no adjustment would need to be made to the curves in order to marble all of the stars, but things get more complicated, as in this second example below:
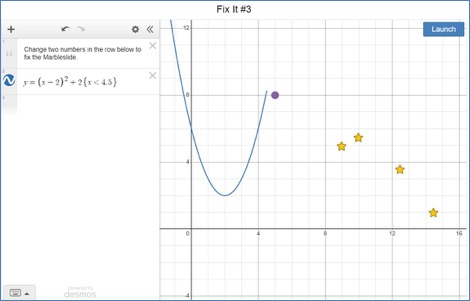
Players would need to make changes to the equation on the left in order to redirect the marbles’ paths. This is gamification at its best! The math is embedded right into the game, rather than in a carrot-and-stick format, where the math is something you have to get right in order to get a reward. In marbleslides, the motivation and the math are on the same team!
Marbleslides games don’t have to involve functions.
Teachers can create (and in fact have already created) versions of marbleslides that are suitable for younger students, for example Marbleslides MiniGolf and Marbleslides Addition, both created by Jenn Vadnais, do not involve functions.
What I loved most about games like this was watching how my students reacted to “success.” It looks very different from how a student reacts to getting back a test and seeing their score. It looks different because it feels different. The test mark reaction is controlled, not particularly empowering, and is over fairly fast. But during my Marbleslides classes I saw students persisting, having aha moments – and sharing them, even high fiving each other, especially of course if they’re working in groups. I can’t say I’ve ever seen students high-fiving each other after getting tests back.
Challenge Creator – where students get to make their own games
Desmos goes one step further with Challenge Creator, in which students can not only play a game, but they can also create their own version of the game for others to play. In Golf Course, by Serge Ballif, students first play golf using angle and distance estimation:
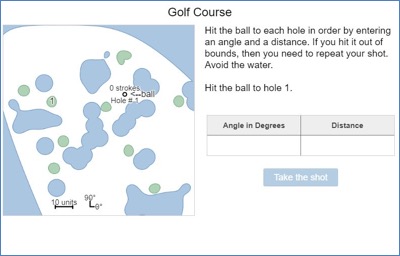
Once they’re successful, they are invited to create their own golf course, with holes and water hazards at the ready. This gives students the opportunity to express their brilliance in a whole new way. They also get to become the teacher in a sense, and experience the thrill of watching someone else solve your own creation. After all, why should teachers have all the fun?
For that matter, why should students have all the fun? As mentioned before, play is a great way to blur the lines between teacher and student. If we join in, say, a polygraph game as a guesser, or try out a golf course that a student created, we have an opportunity to model problem-solving, resilience , and even good mental health practices in dealing with being wrong, or navigating uncertainty.
Conclusion
As much as I like to imagine how I would be a different person had I experienced some of the play described here, I’m even happier imagining its impact on current and future classrooms. Classrooms in which not only is everyone learning, having fun and being creative, but in which there are neither “math people” nor “non-math people”, but simply people. Desmos makes that a real possibility!
Resources
Building Thinking Classrooms in Mathematics, Grades K-12: 14 Teaching Practices for Enhancing Learning by Peter Liljedahl
https://www.goodreads.com/en/book/show/53484824-building-thinking-classrooms-in-mathematics-grades-k-12
Photo credits:
Featured image from Pixabay





